<<
Back to Kanarev's Physchemistry Book Index
11.4. Power of Chemical Bonds of Water Molecules
Let us consider energy content of chemical bonds of the atoms
and the ions being shaped during water formation. Let us assume that we have
managed to begin the formation of water molecules if free protons, electrons
and oxygen atoms are available. Let us calculate quantity of energy released
during the fusion of one litre of water.
There are the
notions of gram-atom and gram molecule
in chemistry. Gram-atom is equal numerically
to atomic mass of the substance, gram molecule is equal numerically to
molecular mass of the substance. For example, hydrogen gram molecule in water
molecule ![]() is equal to 2 grams,
and gram-atom of oxygen atom is equal to 16 grams. Gram-molecule of water is
equal to 18 grams. Thus, one litre of water contains 1000/18=55.56 gram molecules
of water.
is equal to 2 grams,
and gram-atom of oxygen atom is equal to 16 grams. Gram-molecule of water is
equal to 18 grams. Thus, one litre of water contains 1000/18=55.56 gram molecules
of water.
As the mass of
hydrogen ![]() in water molecule
in water molecule ![]() is 2×100/18=11.1% and
the mass of oxygen atom
is 2×100/18=11.1% and
the mass of oxygen atom ![]() is 16×100/18=88.9%, the
ratio between quantity of hydrogen and oxygen is preserved in one litre of
water as well. It means that 1000 grams of one litre of water contain 111 grams
of hydrogen molecules and 889 grams of
oxygen atoms
is 16×100/18=88.9%, the
ratio between quantity of hydrogen and oxygen is preserved in one litre of
water as well. It means that 1000 grams of one litre of water contain 111 grams
of hydrogen molecules and 889 grams of
oxygen atoms ![]() .
.
One litre of
molecular hydrogen ![]() has mass
of 0.09 grams, one litre of molecular
oxygen
has mass
of 0.09 grams, one litre of molecular
oxygen ![]() has mass
of 1.43 grams. It means that from one litre of water it possible to produce
111/0.09=1222.2 litres or 1222.2/22.4=54.56 moles of molecular hydrogen
has mass
of 1.43 grams. It means that from one litre of water it possible to produce
111/0.09=1222.2 litres or 1222.2/22.4=54.56 moles of molecular hydrogen ![]() and
889/1.43=621.67 litres or 621.67/22.4=27.75 moles of molecular oxygen
and
889/1.43=621.67 litres or 621.67/22.4=27.75 moles of molecular oxygen ![]() .
.
Let us pay
attention to the fact that mass of hydrogen atom ![]() is half mass of
hydrogen molecule
is half mass of
hydrogen molecule ![]() . As molecular volume of
all gases is similar and is equal to 22.4 litres, it means that one
litre of water contains 111/0.045=2444.4 l or 2444.4/22.4=109.12 moles of
atomic hydrogen [109].
. As molecular volume of
all gases is similar and is equal to 22.4 litres, it means that one
litre of water contains 111/0.045=2444.4 l or 2444.4/22.4=109.12 moles of
atomic hydrogen [109].
Hydrogen atom
fusion is the process of junction of
free proton with free electron. Energy of the photons emitted by the electron
depends on energy level, on which the electron is held. If it is held on the
first, non-exited energy level, prior to it the electron emits a set of the
photons with energy of 13.6 eV, which is equal to the energy of hydrogen
atom ionization. If the electron is held on the second level, energy of a set
of the emitted photons is 3.4 eV; if it is held on the third level, energy is
equal to 1.5 1itres eV; if it is held on the fourth level , energy is equal to
0.85 eV, etc. (s. Table 29).
The analysis shows
that the electron of hydrogen atom can be on the first non-exited energy level
only in the case if there are no external existing factors in the form of
variables of the electric fields. If external disturbance is constantly present,
the electron in the atom begins to move
from one energy level to another energy
level. In this case energy of the emitted photons and the absorbed ones will
correspond to interlevel transitions of the electron [109].
Now let us consider
the fusion process of water molecule fusion. It begins from the formation of
hydrogen atom. When the electron is bound with the proton, it will try to
occupy non-exited energy level and will emit the photons with total energy of
13.6 eV, equal to ionization energy of hydrogen atom. If we convert this energy
into kilojoules (kJ), we shall have [109]
![]() (281)
(281)
When one mole of atomic
hydrogen is formed, energy is released
![]() (282)
(282)
At the temperature below 4500…5000°C hydrogen
atoms unite in molecules. As chemists
think, energy released during this process is equal to 436 kJ per mole. When
hydrogen molecule unites with oxygen atom, water molecule is formed with the
release of energy of 285.8 kJ per mole. If one treats the above-mentioned
values of energy being released consequently during the fusion of hydrogen
atom, hydrogen molecules and water molecules, the following quantity of energy
will be released [109]
![]() (283)
(283)
![]() (284)
(284)
![]() (285)
(285)
If we sum up the
results being obtained, we’ll have 182832.69 kJ per litre. It is potential
energy, which can be released during the above-mentioned consequent fusion of
one litre of water. If we take into account the fact that energy content of one
litre of gasoline is 30000 kJ, it is 6 times less than the energy released
during the formation of chemical bonds of one litre of water molecules
beginning from the formation of atomic hydrogen [109].
Hydrogen mass
obtained from one litre of water is equal to 1222.2×0.09=109.998 g. Energy content
of one gram of molecular hydrogen is equal to 142 kJ, and energy content of
hydrogen produced from one litre of water is 142×109.998=15619.72 kJ. It is
half of energy content of one litre of
gasoline.
If hydrogen atoms were united in molecules being on the first energy
levels, 182832.69 kJ per l of energy would be released during the fusion of one
litre of water. But everything points out to the fact that hydrogen atoms unite
in molecules only in exited state when their electrons occupy higher energy
levels. A light zone to the right of a bright band on the spectrogram proves it
(Fig. 82) [109].

Fig. 82. Spectral
line (light line to the left) of the second energy level of hydrogen level with
the light continuos zone (to the right from the line) being photographed by
E.D. Zykov from plasma of the plasma – electrolytic reactor.
The bright band itself corresponds to energy
of the photons emitted by the electrons during the transit from the third
energy levels to the second energy levels, and the light zone to the right of
this band corresponds to the spectrum of molecular hydrogen and demonstrates
that the electrons of hydrogen atoms occupy higher energy levels before the
formation of its molecule. As prior to the unification in molecules hydrogen
atoms are in exited state, the energy of this state should be taken into
account during the fusion of the atoms and later on of hydrogen molecules and
water molecules. But we do not know a number of energy level of the electron in
hydrogen atom yet, at which it unites with the neighbouring atom and forms
hydrogen molecule.
One spectral line
of hydrogen atom, which corresponds to the transition of the electron from the
third energy level to the second one, is shown in the spectrogram (Fig. 82, to the left).
Energy of the photon emitted by the electron during this transition is equal to
![]() =(12.0.87-10.198)=1.89 eV. The bright zone to the right from
the band is the molecular spectrum of hydrogen [109].
=(12.0.87-10.198)=1.89 eV. The bright zone to the right from
the band is the molecular spectrum of hydrogen [109].
We should note that
the electrons of the atoms and the ions form on the spectrogram the distinctive
bands, which correspond to the fixed energy levels. The molecules form the so
called striped spectra, which are often merged into continuous light zones
[61], [62]. These are unfixed energy levels. Energies corresponding to fixed energy
levels of the atoms and the ions are determined with great accuracy. Energy of
unfixed energy levels formed by the
electrons of the atoms connected in molecules is changed in definite ranges. In
some cases it is possible to determine an average value of energy corresponding
to some ranges. For example, chemists have determined that 436 kJ are released
during the fusion of one mole of hydrogen molecules. Let us try to determine,
at which energy levels the electrons in hydrogen atoms are arranged before they
are united in a molecule. Let us calculate the energy corresponding to chemical bonds in hydrogen molecule [109]
![]()
As calculated per
atom, it is equal to 4.53/2=2.26 eV. It is binding energy between the atoms in
hydrogen molecule. It is not difficult to calculate [53] that 2.55 eV of energy
are released during the transit of the
electron of hydrogen atom from the fourth energy level to the second one.
If one takes into account that the
spectrum of hydrogen molecule (the light zone to the right from the bright band
in Fig. 82)
is formed in the zone before the second energy level, i.e. in the zone with
energy, which is a bit less than energy of 2.55 eV, there is every reason to consider
that prior hydrogen molecule formation the electrons of its atoms are on the
fourth fixed energy levels. Binding energy of the electron with the protons is
equal to 0.85 eV (Table 29).
Now let us give a
variant of the calculation of energy, which is released during the fusion of
one litre of water being the nearest one to reality. It corresponds to the case
when the electrons of hydrogen atoms being born are kept at the fourth energy
levels and then they are united into molecules. In this case during the
formation of one hydrogen atom energy
of (13.598-12.748)=0.85 eV is released, or in the calculation per mole [109]
![]() (286)
(286)
Then the equation
(286) gives this
quantity of energy (82.0 x 109.12)=8947.84 kJ per litre, and the total quantity
of energy is (8947.84+23788.16+15879.09)=48615.1 kJ per litre during the fusion
of one litre of water. It is more than burning of one litre of gasoline (30000
kJ) or hydrogen (15879.09 kJ) obtained from one litre of water.
Thus, the variant
of the fusion of hydrogen molecules is the most probable at the moment when the
electrons of hydrogen atoms are on the fourth energy levels. In this case
during the fusion of 1 litre of water energy is released, which is
(48615.1/30000=1.62) 1.62 times greater than energy produced during burning of
one litre of gasoline and is (48615.1/15895.15=3.0) 3.0 times greater than
energy produced during burning of hydrogen produced from one litre of water.
Thus, in order to
produce additional energy it is necessary to synthesize at first hydrogen
atoms, then the molecules. The processes of their fusion are the main sources of additional energy.
In an ideal case in
order to check these calculations it is necessary to take free protons, to
connect them with free electrons and to obtain atomic hydrogen and then
molecular hydrogen. Then it is necessary to unite molecular hydrogen with
atomic oxygen and to produce water. When energy released during the fusion
process of hydrogen atoms, its molecules and water molecules is measures, it is
possible to determine, which calculation method reflects reality more exactly.
But it is difficult to carry out such ideal process. It is easier to find an
economical way of water molecule distraction and to obtain additional energy
with the help of its fusion in the above-mentioned sequence.
Now we see that
additional energy is generated by the electrons. Where do they take it from?
When we have considered the model of the electron, we have found out that it
can exist in free state only in case when its electromagnetic mass is strictly
determined. When it is combined with the atomic nucleus, it emits a part of
energy in the form of the photons, and its electromagnetic mass is reduced. But
stability of its state does not become worse, because energy taken by the
photon is compensated by binding energy of the electron with the atomic
nucleus. When it is separated from the atom and becomes free, it should restore
its mass, which corresponds to its free state, in order to maintain its
stability. Where does the electron take it from? The source is the only one -
the physical environment in the form of ether. From this environment, it restores
the energy (mass) being lost in the form of emitted photon. When the electron
restores the constants (mass, energy, charge), it acquires stable free state.
When the conditions for the electron’s
entry into bond are formed, it begins to emit energy in the form of the photons
at once, which corresponds to binding energy. In a new stage of free state, it
restores its constants (mass, charge, energy) again absorbing ether from the
environment. Thus, the electron transforms energy of the environment into energy
of the photons [109].
We have already published this hypothesis in one of our articles
printed in USA [72]. It is published in Russian for the first time. A question arises: is free space available
in the atom, which can serve as a source of ether absorbed by the electron in
the process of restoration of its constants? The answer can be got from the
geometrical parameters of the atom, and they are as follows: if the size of the
atomic nucleus were equal to one mm, the size of one electron in the atom would
be nearly one metre, and the size of the atoms itself would be nearly 100
metres. It means that the atoms has enough space filled with ether, which is
necessary for the electron for the restoration of its constants after the bond
with an atomic nucleus or with the
electron of the neighbouring atom is lost.
It means that physical vacuum is a source of additional
energy and the electron is a converter of energy of vacuum into energy of the
photon [84].
The given results of calculations and experiments demonstrate
the possibility of production of
additional energy during water electrolysis, but for this purpose it is
necessary to create the conditions for the implementation of this possibility.
The preliminary analysis of manifestation
of additional energy in the phenomena of water cavitations shows that the
source here is the same as during water electrolysis. Mechanical destruction of
water molecules leads to the further fusion of the atoms and the molecules of
hydrogen and water. The electrons play here the same role as during water
electrolysis. They transform energy of vacuum into energy of the photons.
11.5. Clusters and
their Binding Energies
It is known that
water molecules can be units with each other forming the associations called
clusters. The clusters are a set of the molecules of the same name, which
are connected with each other by hydrogen
bonds, as it has been considered earlier. And it is so indeed. Water
molecules connect the protons of the hydrogen atom into clusters (Fig. 83).
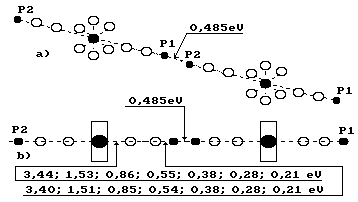
Fig. 83. Diagram of
a cluster made of two water molecules
Now we can call them electron bonds,
proton bonds or electron - proton bonds. The chemical formula of the cluster
consisting from ![]() of ions of
of ions of ![]() and water molecules is put down in the following
way [48]
and water molecules is put down in the following
way [48]
![]() (287)
(287)
If ion ![]() participates, the
reaction takes place in such a way
participates, the
reaction takes place in such a way
![]() (288)
(288)
There are
experimental data of binding energies between water molecules and ions ![]() and
and ![]() when their different
quantity is present in a cluster (Table 37) [48].
when their different
quantity is present in a cluster (Table 37) [48].
Table 37. Values of
binding energy in clusters, eV
|
Value n |
0-1 |
1-2 |
2-3 |
3-4 |
4-5 |
5-6 |
6-7 |
|
H3O+ (H2O)n |
1.56 |
0.97 |
0.74 |
0.67 |
0.57 |
0.51 |
0.45 |
|
OH-(H2O)n |
1.1 |
0.71 |
0.66 |
0.62 |
0.61 |
- |
- |
For example, 5.47
eV are consumed for the formation of cluster ![]() when n=7.
when n=7.
The process of the
formation of clusters is an endothermic one, i.e. during the formation of the
clusters the electrons, which unite the molecules with each other, move off
from the atomic nuclei in their cells.
Having the
structure of water molecule (Fig. 72-74) we see other possibilities of cluster formation.
There are no restrictions for the formation of proton-to-proton bonds between
water molecules. The protons of the first hydrogen atoms in two water molecules
uniting with each other form the association of two molecules (Fig. 83). The protons of
the second hydrogen atoms in water molecules as well as the first and,
probably, the second electrons of oxygen atoms can be involved in this process.
As a result, the number of molecules in the cluster will be increased.
Thus the presence
of the ions of hydroxyl and hydroxonium is unnecessary for the formation of the
clusters of water. Let us consider the structure of parahydrogen molecule in
Figs 53, b and 80, c. It can be a
connecting link in the cluster, and after its destruction hydrogen can be born
at once not in the atomic state, but in the molecular one.
If under usual
conditions water molecules are united in the associations known as clusters,
binding energy between clusters moves to zero during the conversion into vaporous
state, and we have the possibility to calculate binding energy between the
molecules in the cluster at the temperature of 20°C. We’ll use vapour formation
energy of 2595.2 kJ per kg for this purpose. Let us convert this energy into
electron – volts in the calculation per molecule (Fig. 83).
![]() (289)
(289)
This result is close to binding energy (0.54 eV) of the
electron of the first hydrogen atom in water molecule when it is on the fifth
energy level (Table 35) and proves that the proton of this atom spends the
majority of magnetic line of force for the bond with the electron, and the
minority is free and can be used for the bond with the proton of hydrogen atom
of the neighbouring water molecule (Fig. 83). One more problem takes
place here, about which we’d like to say some words.
What law controls
the alternation of the magnetic poles
of the protons in atomic nuclei? The reader understands that the answer to this
question can be given by a new book, and not only by one book, that’s why we
give a short answer, which seems to be obvious. It is impossible that the south
or north magnetic poles of all protons of the nucleus are directed to its
surface or to the centre. They alternate in such a way that strength of the
nucleus is increased. They are in sequence, and strength of the nucleus is increased. It can
be easily seen that the axial neutrons and protons in the nucleus of the oxygen
atom (Fig. 28) are connected by unlike magnetic poles at different ends of the
nuclei axis and leave different magnetic poles.
It leads
automatically to various magnetic polarity of the protons of hydrogen atoms in
water molecules, and in this case the conditions are formed for
proton-to-proton bond between water molecules and for the formation of clusters
in such a way.
There is every
reason to believe that one and the same proton in various nuclei of atoms can
be arranged in such a way that the north magnetic pole in one nucleus will be
directed to the centre of the nucleus and in other nucleus to its surface. Such
arrangement of the magnetic field of the proton provides the formation of
proton-to-proton bond between water molecules during the formation of clusters.
The atomic nuclei
are the beginning of the formation of various magnetic poles of valence
electrons on the surface of the atoms, that’s why there is every reason to
believe that all atoms are divided into two classes according to this feature,
these classes can be conditionally called «masculine» and «feminine».
If we imagine a
cluster consisting of two water molecules, which have the forms of the balls
with the diameters of 100 metres, the protons arranged on the surface of these
balls and uniting them in a cluster have millimetre dimensions. The smallest,
even mechanical influence will destroy this system creating the conditions for
fluidity of water molecules.
If the clusters had
been formed by electron - to - electron bonds, they would have had metre
dimensions of the surface of one hundred metre molecules.
Now it is possible
to calculate energy spent for heating of one water molecule by one degrees. It
is known that 335.2 kJ of energy is
spent for heating of one litre of water from 20°C to 100°C. Calculating per
molecule it will be
![]() (290)
(290)
It is energy value, by which binding energy of
valence electrons is changed in water
molecule if it is heated from 20°C to 100°C. If we divide 0.063 eV by 80, we’ll get a
value, by which binding energy of valence electrons is changed during water
heating by one degree. It is equal
0.00078 eV. This
energy corresponds to the photons of a
relic range, which covers a part of the infrared range and the microwave
one (Table 34).
Thus, minimal energy of the photons absorbed
by the electrons of water molecules during heating corresponds to the energies
of the photons of the relic range; it serves as an indirect proof that this
range a boundary of existence of single
photons [109].
Now it is possible
to specify energy level number, at which the electrons of hydrogen atoms are in
water molecules. For this purpose let us convert energy (286 kJ) of fusion of
one mole of water in electron - volts.
![]() . (291)
. (291)
It will be
2.97/2=1.485 eV in calculation to one bond. It is near to energy of 1.51 eV of
the third atomic energy level. Later using energy consumption (4 kWh) for the
production of one cubic metre of hydrogen we have found out that binding energy
of hydrogen atom with oxygen atom in water molecule is equal to 1.59 eV. Energy
consumption for heating of water during its electrolysis is included into this
value. If water is heated up to 80°C, binding energy
is equal to (1.59-0.00078x60)=1.543 eV at 20°C. Good approximation of two
results to energy (1.51 eV) of the third atomic energy level of hydrogen atom
points out to the fact that we can trust the fusion energy values of one mole
of molecular hydrogen of 436 kJ per mole and fusion energy of one mole of water
molecule of 286 kJ per mole. The results being obtained show that the electron
of hydrogen atom in water molecule is not on the third atomic energy level
exactly (1.51 eV), but near it.
One cubic metre
contains 1000 x 0.09=90 g of hydrogen. Energy content of one cubic metre of
hydrogen is 142 x 90=12780 kJ. Produced energy of 12780 kJ is equal to
(12780/3600)=3.55 kWh. If one can manage to spend less energy consumption for
the production of one cubic metre of hydrogen than 3.55 kJ, it will be a competitive
energy carrier [109].
The analysis of the
model of the electron (Fig. 18) shows the possibility of the formation of the
clusters of the electrons. The unlike magnetic fields of the electrons bring
them together, and the like electric fields restrict this approach. Accuracy of
this consequence is confirmed by the experiments [185], [186], [188].
The
Foundations of Physchemistry of Microworld
Copyright Ó2003 Kanarev Ph.
M.
Internet Version - http://book.physchemistry.innoplaza.net
<< Back to Physchemistry Book Index