<< Kanarev’s Page

THE DOPPLER EFFECT AT PHOTON EMISSION

 

Ph.M. Kanarev

The Kuban State Agrarian University, Department of Theoretical Mechanics

13, Kalinin St., 350044  Krasnodar, Russia

E-mail: kanphil@mail.kuban.ru

 

 

       Abstract:  It is shown that the Doppler Effect calculation at photon emission is possible only with the help of classical mathematical models.  

         Key words:  The Lorentz transformations, the Doppler Effect, infrared and ultraviolet shift of the spectra, velocity of  light, velocity of source.

Introduction

 

            The results of our investigations confirm Albert Einstein’s correctness in his dispute with Niels Bohr [1], [2], [3]. Einstein’s answer to the venerators of his talent has proved to be a prophetic one: “It seems to them that I look at the results of my life with a halcyon satisfaction. But everything is to the contrary if examined closely. There exists no concept, in relation to which I am sure that it will remain inviolable, and I am not convinced that I am on the right track.” (F. Gernek. Albert Einstein. M., 1966, page 16) [4]. The following facts confirm equity of these words.

There are two interpretations of this effect: the relativistic interpretation and the classical one [3], [5], [6], [7].

 

The Relativistic Interpretation of the Doppler Effect

 

         The relativistic interpretation is based on the second Einstein’s postulate [8]: Any ray of light moves in the stationary system of co-ordinates with the determined velocity, whether the ray be emitted by a stationary or a moving body. The Lorentz transformations originate from this wording of the postulate  (Fig. 1) [2], [3]:

 

 ;                                                                             (1)

 

,                                                                               (2)

 

where:  and  are spatial intervals measured in moving  and stationary  reference systems, respectively (Fig. 1);  and  is time measured in moving and stationary  reference systems, respectively;  is traverse speed of moving reference system;   is velocity of light.

 

 

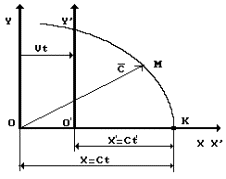
Fig. 1. Diagram to analysis of the Lorentz transformations [2], [3]

 

           It is clear from Fig. 1  that  and  . If we insert these values in the formula (1) or (2), we’ll find

 

                                                                                       (3)

or

 ,                                                                                   (4)

 

        where  and  are frequencies of electromagnetic emission in the moving and stationary reference systems,            respectively.

             If we designate  ,  we’ll have

                                                                            (5)

            This is the relativistic mathematical model of the calculation of the Doppler Effect [3], [8]. As  , it results from the ratios (4) and (5) that emission frequency  of the moving source is greater than radiation frequency  of stationary source, i.e. the mathematical models (4) and (5) describe only ultraviolet shift of the spectra. Let us pay attention to the fact that in the ratios (4) and (5) the directions of velocities of source  and the emitted photon    coincide with the directions of axes  and   .

              If we write the ratio (5) in the following form

,                                                                   (6)

 

it will show to what extent frequency  of the photon emitted from the stationary source (Fig. 1) is less than frequency    of the photon emitted by the moving source, and it will not characterize the infrared shift of the spectra. As in both formulas (5) and (6) , both formulas describe the ultraviolet shift of the spectra, and we have no right to use the mathematical model (6) for the calculation of the infrared shift of the spectra [3].

              Let us prescribe several values  and determine values  (5) and      (6) for them (Table 1).

 

       Table 1

Relativistic results of calculation of the Doppler Effect

 

	(5)	(6)
0.000001 0.00001 0.0001 0.001 0.01 0.1	1.0000009 1.0000099 1.0000999 1.0010004 1.0100504 1.1055415	0.9999989 0.9999899 0.9998999 0.9990004 0.9900494 0.9045340

 

            The results of  Table 1 show unambiguously that frequency  of the emitted photon is increased with the increase of velocity  of movement of the reference system (for example, of a star). It means that an ultraviolet shift of the spectral lines is increased. Physical sense being present in mathematical symbols  and   deprives us of the right to give another interpretation of the mathematical models (5) and (6).

              Thus, we have got an unambiguous answer: the relativistic mathematical models (5) and (6) describe only ultraviolet shift of the spectra, and they are not connected with their infrared shift [5].

              In science, the Lorentz transformations (1) and (2) have been used for the calculation of the so called relativistic effects for about a hundred years. Now we get to know that they give the possibility to calculate the relativistic effects only for the ultraviolet shift of the spectra and provide no information concerning the relativistic effects by the infrared shift of the spectra. It means that the Lorentz transformations misrepresent the reality. Now we can check this fact using the axiom of space-matter-time unity [2], [3].

             Let us pay attention to the fact that formula (1) has coordinate , which is fixed in the moving reference system (Fig. 1), and formula (2) has time , which flows in the same reference system. Thus, in mathematical formulas (1) and (2) an oscillating quantity of the spatial interval  in the moving reference system is separated from time , which flows in this reference system. As in reality it is impossible to separate space from time, it is impossible to analyze these equations separately. This is a set of equations, and it is necessary to analyze them together. This analysis alone will correspond to the axiom of space-matter-time unity, and the results of such analysis alone will reflect reality.

              It results from equation (1) implicitly that by  the spatial interval value  is reduced. Thereof modern physicists make a conclusion that the spatial interval value  is reduced with the increase of velocity  of movement of the moving reference system. Then, they take one equation (2) for the analysis[1]. It appears from this implicitly that by  the spatial interval value  is reduced. Thereof they make a conclusion that if traverse velocity of the moving reference system is increased, flow rate of time  in it is reduced.

               Let us correct erroneous interpretation. As in reality it is impossible to separate space from time, let us analyze equations (1) and (2) together; for this purpose, let us divide the fist one by the second one; as a result, we’ll have

 

                                                                       (7)

 

               Now mathematical formula (7) reflects dependence of coordinate  on time . It appears from this that formula (7) operates within the framework of the axiom of space-matter-time unity, i.e. within the framework of reality.

            It is clear from Fig. 1 that  is the coordinate of position of a light signal in the stationary reference system. If we insert   in the given formula (7), we’ll get coordinate , which positions the light signal in the moving reference system. Where is this signal situated? As we change coordinates  and , in time  and  it is situated on coinciding axes  and   , i.e. in point  (Fig. 1). Geometrical sense of  the Lorentz transformations is very simple. Coordinate  of point  in the moving reference system and its coordinate  in the stationary reference system are positioned in them. It is a crossing point of a light sphere with axes  and  (Fig. 1). This is the essence of the Lorentz transformations. There is no other information in these transformations, and they reflect no physical effects [2], [3].

 

Classical Interpretation of the Doppler Effect at Photon Emission

 

          We have already shown that total energy of the photon is equal to the sum of energies of its translational motion  and rotational motion  and that this amount depends on the value of speed  and direction of radiation source motion  [1], [2], [3]. If the angle between the direction of velocity vector of source motion  and the direction of velocity vector of the photon being emitted (Fig.2) is equal  , total energy of the emitted photon will be written in the following way [7].

 

 

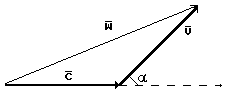
Fig. 2. Diagram of addition of velocities of the source and the photon

 

                         (8)

 

If we take into account that    and designate   , we’ll find after conversion of the equation (8)

 

                                                  (9)

 

If the directions of the motion of the source and the photon being emitted coincide, it means that  and

                                                             (10)

 

          When the directions of the motion of the source and the photon being emitted are counter, it means that and

                                                       (11)

 

           In Table 2, the calculation results are given according to the classical formulas (10) and (11) and relativistic formulas (5) and (6). Analysis of this table shows that the classical formula (10) gives the result, which is close to the result of the relativistic formula (5), and classical formula (11) gives the result, for which there is no relativistic formula,

Relativists use the formula (6) for the calculation of the infrared shift of the spectra having no mathematical right for it. Such right and such accuracy are given by the classical mathematical formula (11) (Table 2).

Table 2

Calculation results of Doppler Effect

 

 

              The results of the simultaneous registration of the usual spectral lines of the hydrogen atom received from the space object SS433 and the spectral lines shifted to the ultraviolet and infrared field of the spectrum can serve as an experimental fact, which confirms equity of mathematical models (10) and (11) [9]. It proves the fact that the main part of the space object SS433 is stationary in relation to space, and two other parts move in relation to space. The part, which generates the ultraviolet shift, moves to the Earth, and the part, which generates the infrared shift at that time, moves from the Earth. Periodicity of the change of values of these shifts is registered [9].

 

CONCLUSION

 

        The relativistic mathematical model (6), which originates from the Lorentz transformations, has nothing to do with the Doppler Effect.

         The ultraviolet and infrared shifts of the spectra describe the classical mathematical models (10) and (11) only.

 

REFERENCES

 

1.        Kanarev Ph. M. Modelling the Photon and Analyzing Its Electromagnetic and Physical Nature. Journal of  Theoretics. Vol. 4-1, February 2002.

        2.    Kanarev Ph. M. On the Way to the Physics of the XXI Century, Krasnodar, 1995, 269 pages (in English).

         3.  Kanarev Ph. M.  The Foundations of  Physchemistry of  Microworld. Krasnodar. 2002. 320 pages (in Rusian and in English).

         4.  Hernek F. Albert Einstein.  Life for Truth, Humanism and Peace.  M.: Progress. 1966.

         5.   Macarthur D.W., Butterfield K.B., Clark D.A., Donahue J.B. and Gram P.A.M., Brgant H.C., Smith W.W. and      Comtet G. Test of the Special Relativistic Doppler Formula  at  . Physical Review Letters. Vol. 56, no. 4, pp. 282-285. 1986.

6.        Pobedonostsev  L. A. Experimental investigation of the Dopler effect. Galilean Electrodynamics. Vol. 3, no. 2, pp. 33-35. (March/April 1992).

7.        L. B. Boldyreva, N.B. Sotina.  The Possibility of Developing a Theory of Light Without Special Relativity. “Galilean Electrodynamics” . Volume13, Number 6. Pag. 103-107.

            8.  Einstein A. To electrodynamics of moving bodies. Collection of articles on special theory of relativity. M.                 Atomizdat. 1973.

             9.   Chernin A.D. Stars and Physics. M. Nauka, 1984. 160 pages.